This is not a Quibans about politics (and when I use this with my class I won’t be taking about the politics), but it is about common mathematical/statistical errors that people make.
It is unusual in that it starts with a tweet. I don’t usually create a Quibans based on a ‘random
person tweeting’, but because this came from the account of a former MEP who
has over 70,000 followers, it seems reasonable to do so.
With my class I will start by showing the following
images. Here’s the first tweet:
This image was part of that tweet:
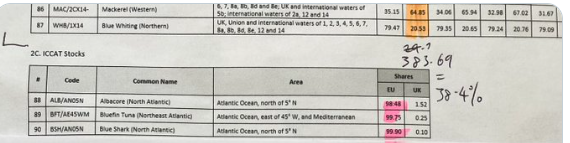
Here’s part of an image from one of the other tweets in the thread:
I am anticipating some of the following:
1) Where do the numbers come from?
2) What do they mean?
3) What is he working out?
4) Why is he doing it ‘by hand’?
And here are my responses:
1) This is from the ‘Annex’ to the Brexit Trade Deal that was agreed by the EU and the UK government on 24 December and which is due to be voted on by MPs before the end of 2020. This is a printout of pages 893 and 894.
2) It shows the percentage of each type of fish that is allowed to be caught in different areas of sea by UK and by EU fishing boats.
3) The two orange highlighted columns show the percentage for UK boats in 2021 and in 2026. He is working out the average of these for each page.
4) He has presumably used a calculator to add them up and then to divide by the number of types of fish on the page. Why hasn’t he just copied it into a spreadsheet? The document it is in is a pdf, and the annex is an image, so the text can’t just be copied and pasted! I have retyped those two columns (I think I have done it accurately). They are available on this spreadsheet. (The spreadsheet hasn’t been optimised for printing. Pages 1 to 4 include a screenshot of the original document and my typed version of the relevant columns. The final sheet includes all of the typed data.)
Tasks for the students:
A) For page 1, calculate the total and the mean to check the figures he has worked out here. How many different ways can you work out the mean?
B) Now do the same on page 2. What is going on here? (Find the error!)
D) Go to the final sheet. What is the mean for all of the fish for 2021? What is it for 2026?
E) Why is it not reasonable to say there is a 2.32% increase? Using his figures and his methodology, what would be a more sensible value to give for the increase?
F) If UK boats catch 20% of a type of fish in one year and 30% the next, why might they not be landing more fish in the second year? What information is missing here?
G) Why can’t we find the mean of the 2021 figures and the mean of the 2026 figures and then calculate the change? (This is a major issue!)
Some answers:
A) This is a nice opportunity to use Excel in different ways. The calculations for page 1 are correct. (He has sensibly rounded the answers for the mean.) We can use =SUM( : ) to work out the total and can then divide that answer by 24 (there are 24 types of fish stocks on the first page) or can do =AVERAGE( : )
B) On page 2 the total for 2026 is correct. But he has then divided by 27. This should be 28. If you use the ‘average’ command on Excel you get the correct answer and this is the same as dividing by 28. Why has he got this wrong? The second page starts with number 25 and goes up to number 52. He has done 52 – 25 = 27. This seems an obvious thing to do for lots of people. Why is it wrong? How many ways can they explain it?
[If you count them you find there are 28 of them. Or you could consider that on page 1 it goes from 1 to 24, but that is clearly 24 different fish – you don’t do 24 – 1. Or: if you subtract 25 you are getting rid of fish number 25 – we need to remove fish number 24 (and the earlier ones), so it should be 52 – 24.]
C) Page 4 includes fish number 77 to 87. If he subtracts then he will assume there are 10 fish on that page, whereas it should be 11. The total is 383.69. He will divide by 10 to get 38.37% whereas it should be divided by 11 to give 34.88%. (In fact he rounded off to 1dp this time and got 38.4%) Note that you can use SUM and AVERAGE in Excel across the blank cells and cells with text in them – it just ignores them and still gives the correct answers.
D) For 2021 the mean is 33.47% and for 2026 it is 35.93%. These are close to the values he gives (of 33.6% and 35.92%) but not identical. In fact, in the tweet he writes about 2020 and not 2021. I tweeted to ask about this, but didn’t receive a reply.
E) He seems to be saying that because the UK share (according to his calculations) is going from 33.6% to 35.92% then that is an increase of 2.32%. We ought to refer to this a an increase of 2.32 percentage points. From the perspective of the UK fishing industry, the percentage is going from 33.6 up to 35.92. This is an increase of 2.32 over the original value of 33.6. That’s actually an increase for the UK boats of 6.9%.
F) The document talks about the percentage of fish that can be caught by the two fishing fleets. It doesn’t confirm that the total amount is the same each year. If 10 tonnes can be caught one year and 1 tonne the next, then 20% of 10 tonnes is 2 tonnes (2000kg) whereas 30% of 1 tonne is 300kg. (I couldn’t find any figures about the total quotas, so I have no idea whether the amounts are the same each year.)
G) There is a massive issue here, which means all of the information in the original tweet (and everything that has happened so far in the earlier tasks) is just nonsense. We cannot find the average of the percentages in the way the former-MEP did, because they are likely to be percentages of different amounts. Here’s a simplified example. If the UK fleet gets 10% of Fish A and 20% of Fish B, it doesn’t necessarily get 15% of all of the fish. Suppose 100 tonnes of Fish A is landed in total and 1 tonne of Fish B is landed then 10% of A is 10 tonnes and 20% of B is 0.2 tonnes. Altogether the UK boats get 10.2 tonnes out of 101 tonnes, which is 10.1%. Or if the UK fleet has 100% of Fish A and 0% of Fish B then it gets 100/101 = 99% of the fish and not the average of 100% and 0%.
Any time you see averages of averages it is worth asking
whether it is fair/reasonable to do this!
Sources: https://twitter.com/MartinDaubney/status/1343147201112010753